September 23 2017at 1159 pm. The z-transform is useful for the manipulation of discrete data sequences and has acquired a new significance in the formulation and analysis of discrete-time systems.
Ghulam Muhammad King Saud University The z-transform is a very important tool in describing and analyzing digital systems.

Z transform mathematics pdf. If you know what a Laplace transform is Xs then you will recognize a similarity between it and the Z-transform in that the Laplace transform is the Fourier transform of xte t. Inverse Z-Transform Transform from -domain to time-domain Note that the mathematical operation for the inverse z-transform use circular integration instead of summation. Although motivated by system functions we can define a Z trans.
It offers the techniques for digital filter design and frequency analysis of digital signals. Z-TRANSFORMS 41 Introduction Transform plays an important role in discrete analysis and may be seen as discrete analogue of Laplace transform. Most useful z-transforms can be expressed in the form P z X z Q z where P z and Q z are polynomials in z.
Zero c k zeros of Xz. Region of convergence ROC. Ran a n1 a 1a PRan Pa 1an.
Cas Lft Z 1 0 estftdt for s 2 C. If a system is stable then y hn 0 for all initial conditionsIn this case we call y h the transient. 1803 Di erence Equations and Z-Transforms 6 Transient.
If is a casual sequence ie if for n0then the Z-transform is. Z - Transform 1 CEN352 Dr. Note capital letter for transform.
The z-Plane Poles and Zeros 8 To represent z rej graphically in terms of complex plane Horizontal axis of z-plane real part of z. It is a mapping from the space of discrete-time signals to the space of functions dened over some subset of the complex plane. Similarly for a given z-transform Xz there exists one and only one sequence fxng1 n0 for which the series in 51converges for jzjR.
X z x n z. With the Z-transform. Role of Transforms in discrete analysis is the same as that of Laplace and Fourier transforms in continuous systems.
We define the Laplace Transform of a function f. Relation between DTFT and z-transform. Reveals that the Z-transform is just the DTFT of xnr n.
1 2𝜋 1. Thanks a lot mam. Is a complex variable.
The values of z for which the. Form for any signal. N n Notice that we include n.
However for discrete LTI systems simpler methods are often sufficient. Z- Transform and Applications z-Transform is the discrete-time equivalent of the Laplace transform for continuous signals. The DTFT is given by the z-transform evaluated on the unit circle pole.
If is a sequence defined for then is called the two-sided or bilateral Z-transform of and denoted by or where z is a complex variable in general. This is due to the continuous value of the z. Z-transform plays an important role in analysis of linear discrete time signals.
In this thesis we present Z-transform the one-sided Z-transform and the two-dimensional Z-transform with their properties finding their inverse and some examples on them. For example one can invert the. The z-transform definition involves a summation 2.
In the math literature this is called a power series. Hope you will be making notes for next 2 units of 3rd sem also. Sum converges define a region in the z-plane referred to as the.
See below for the extended version of this theorem. Xz and its associated region of convergence. 3 The inverse z-transform Formally the inverse z-transform can be performed by evaluating a Cauchy integral.
31 Inspection method If one is familiar with or has a table of common z-transformpairs the inverse can be found by inspection. Very nice compilation of material and giving it yours touch of writing really make these notes fruitful to students who bunk there classes in exam time. Substituting z rej in the Z-transform Xz X1 n1 xnr ne jn.
H z h n z. Where z re. The z-transform plays a similar role for discrete systems ie.
The zeros and poles completely specify X z to within a multiplicative constant. A differential equation will be transformed by Laplace transformation into an algebraic equation which will be solvable and that solution will be transformed back to give the actual solution of the DE we started with. Z-transform is used in many areas of applied mathematics as digital signal processing control theory economics and some other fields 8.
Notationally if x n has a z-transform X z. Ones where sequences are involved to that played by the Laplace transform for systems where the basic variable t is continuous. 51 z-Transform and its Inverse.
DefinitionThe z-transform of a discrete-time signal x n is defined by. 0 bilateral Z transform there is also a unilateral Z transform with. Vertical axis of z-plane imaginary part of z.
The values of z for which P z 0 are called the zeros of X z and the values with Q z 0 are called the poles. 0 as well as n. A solution to PRy an is yn an Pa 1 Proof.
The uniqueness for the z-transform derives from properties of power series expansions of complex functions of complex ariablesv. Z transform maps a function of discrete time. The z-transform and Analysis of LTI Systems Contents.
The z-transform converts certain difference equations to algebraic equations. We will also call the complex plane the z-plane. It is used extensively today in the areas of applied mathematics digital signal processing control theory population science economics.
To a function of. It is seen as a generalization of the DTFT that is applicable to a very large class of signals observed in diverse engineering applications.

Transformee De Laplace Tableau

Z Transforms And Difference Equations

Region Of Convergence For The Z Transform Youtube

Pdf Table Of Laplace And Z Transforms Eyob Alemayehu Academia Edu

Z Transforms And Difference Equations
Transformee De Laplace Tableau
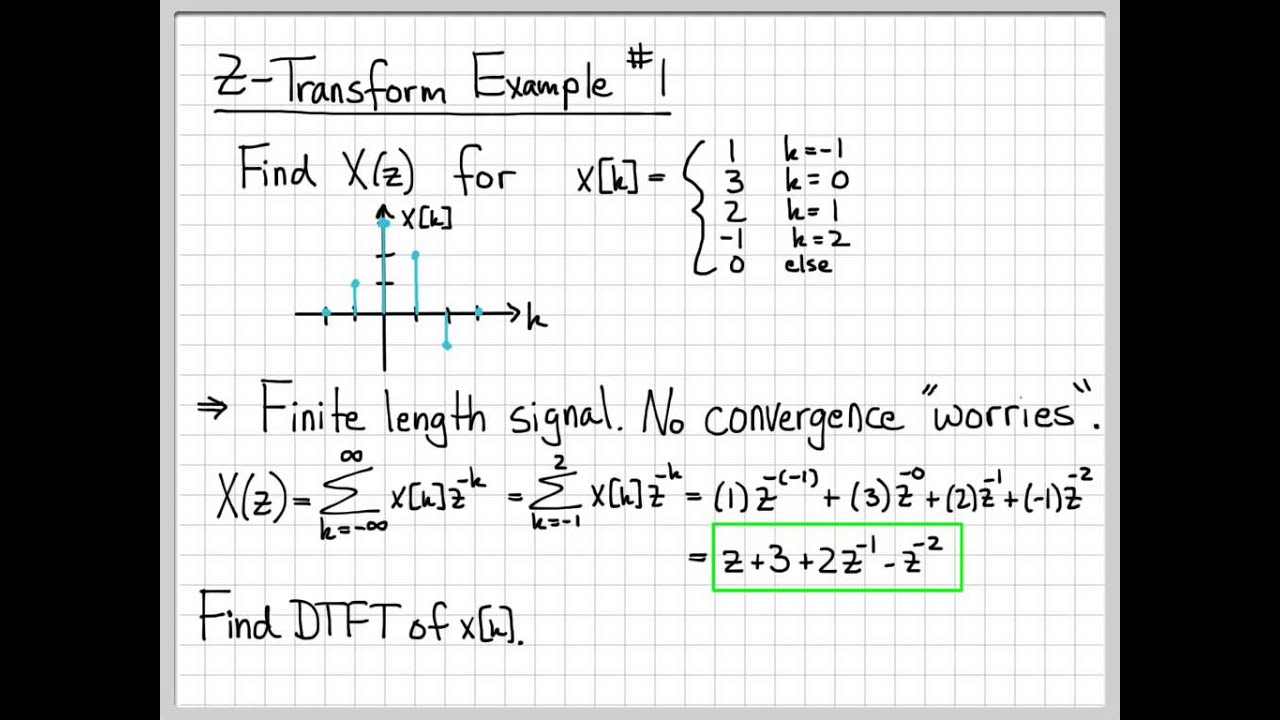
Z Transform Example 1 Z Transform Part 1 Youtube


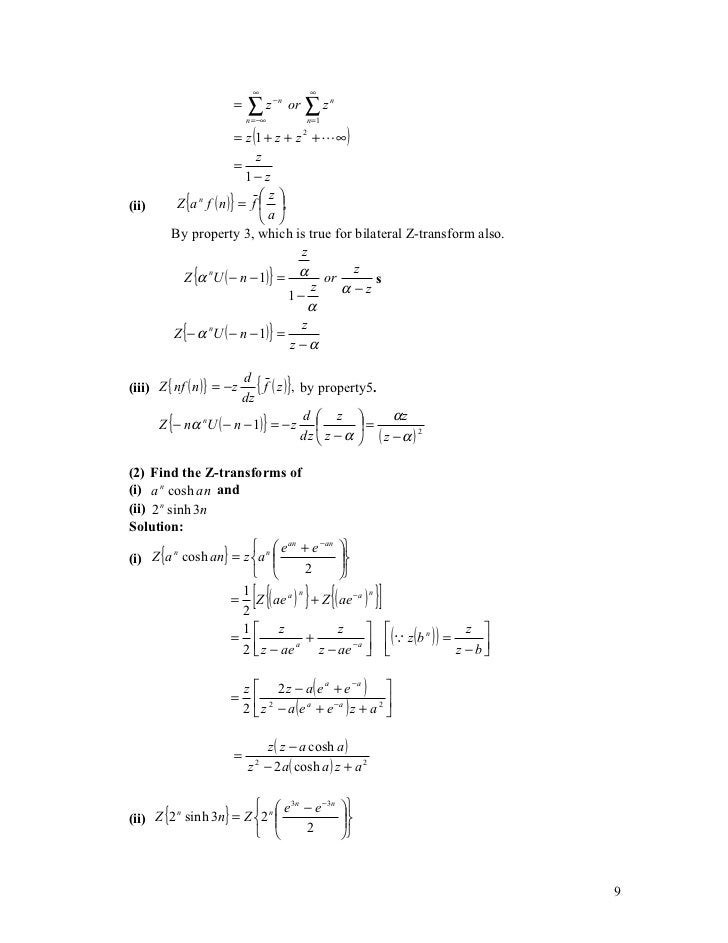

0 comments:
Post a Comment